Due to the skin effect in the microwave band, the current in the conductor of the microwave device is usually concentrated in the thickness of the surface in micrometers, which is a well-known concept. According to this principle, the industry generally adopts the following methods to improve the loss of microwave devices, and at the same time reduce the cost of the device: deposit a good conductor layer with a skin depth of several skin depths (such as gold plating and silver plating) on ​​the surface of a conductor (such as aluminum alloy). . This principle also applies to improving the passive intermodulation performance of microwave passive devices: low intermodulation devices usually use silver-plated surfaces.
In order to measure the loss properties of conductor materials, the concept of surface resistance R is defined:
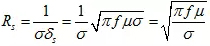
In other words, the surface resistance is inversely proportional to the product of the conductivity and the skin depth. Based on this concept, device losses can be easily evaluated. Taking a rectangular waveguide as an example, if only the loss of the broadside of the rectangular waveguide is considered, the loss of the waveguide transmission line can be calculated as follows if operating under the fundamental mode TE10:
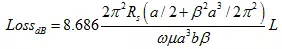
Phase constant calculation method:
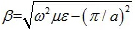
The image understanding of the skin depth is: Since the electromagnetic wave exhibits an exponential decay inside the good conductor, when the electromagnetic wave penetrates into a thickness of the skin depth inside the conductor, the electromagnetic wave amplitude will be attenuated by 1/e, that is, 36.8%. If the conductor thickness reaches three skin depths and above, it is generally considered that the electromagnetic wave amplitude decays to zero.
The above is our general understanding of the relationship between conductor loss and skin depth. The following discussion will address the question: If the thickness of a good conductor equals the skin depth or even less than the skin depth, will the electromagnetic wave penetrate the good conductor? The above mentioned “surface resistance equals the inverse of the product of the conductivity and skin depth†should be used when the conductor thickness is less than the skin depth.
In fact, the discussion of skin depth in textbooks is based on an ideal model of infinite conductor thickness. When the conductor thickness equals the skin depth or even far smaller than the skin depth and the conductive film evolves, the electromagnetic wave penetrates into the interior of the conductor, and emission occurs due to a mismatch between the conductor film impedance and the air resistance when the conductor film is exited (assuming that the conductive film is reversed. For air media). Therefore, even if the thickness of the conductive film has only one skin depth, the attenuation of the electromagnetic wave is no longer 36.8% as described above.
According to AE Kaplan's paper “on the reflecTIvity of metallic films at microwave and radio frequenciesâ€, in the free space, when the electromagnetic wave is perpendicularly incident on the conductive film, its power transmission coefficient can be calculated as follows:
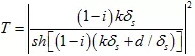
The following figure shows the effect of aluminum thickness on the attenuation of electromagnetic waves based on this theory (conductivity 38.6 MS/m, frequency 10 GHz).
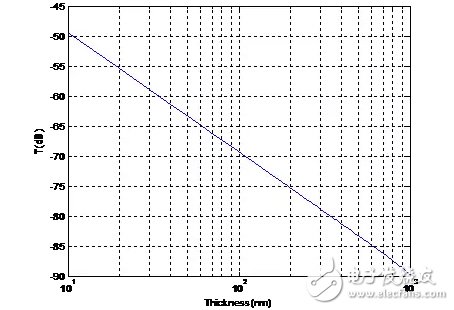
Figure 1. Electromagnetic wave attenuation by conductive films
From the above figure, even if the thickness of the aluminum film is only 10 nm, its attenuation to the 10 GHz electromagnetic wave can reach 50 dB, which is far greater than the attenuation that we usually understand from the perspective of skin depth.
In fact, in the case of conductive films, the surface resistance can be expressed as:
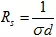
That is, assuming that the wall thickness of the rectangular waveguide is smaller than the skin depth, when calculating the conductor loss, the skin depth in the surface resistance formula in the textbook needs to be replaced with the film thickness. Below, we give the simulation results of HFSS, and compare with the theoretical calculation results. The electromagnetic waveform is shown in Fig. 2. Similarly, only the loss of the broadside of the waveguide is considered here. The simulation results are shown in Fig. 3 (the loss term in the figure represents the radiation loss; the black line corresponds to the left ordinate, and the red line corresponds to the right ordinate): when the conductivity is 0.5 MS/m, the radiation loss is significant and the film is The thickness increases and decreases; and when the conductivity is 30 MS/m, the radiation loss significantly decreases.
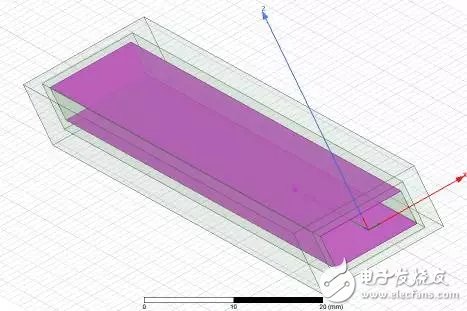
Figure 2. Rectangular Waveguide Model with Conductive Thin Films on Wide Edges
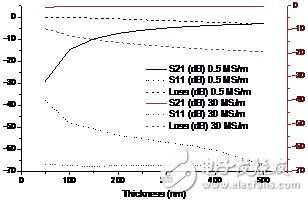
Figure 3. Simulation results of the effects of conductivity and film thickness on S-parameters and radiation losses of rectangular waveguides
Figure 4 shows the comparison between the HFSS simulation and the theoretical calculation results: The theoretical calculation results using the surface resistance defined by the conductivity and film thickness are in good agreement with the HFSS simulation results, indicating that the surface resistance should be the conductivity in the case of conductive thin films. The inverse of the product of film thickness.
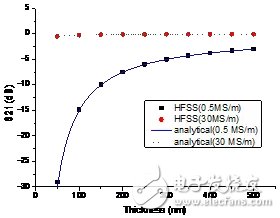
Figure 4. Comparison between HFSS simulation and theoretical calculation
The main conclusion: if the thickness of a good conductor is equal to or even smaller than the skin depth, the electromagnetic wave will still penetrate the good conductor, but it is much smaller than the attenuation that we usually understand from the perspective of the skin depth; the surface resistance is equal to the conductivity and The inverse of the skin depth product" should be replaced by "the surface resistance equals the reciprocal of the product of the electrical conductivity and the film thickness."
This is a special maskking High 2.0 e-cigarette product series. We sell maskking high 2.0 vape, maskking 1000 puffs, and other pod maskking high 2.0.
We are specialized electronic cigarette manufacturers from China, Vapes For Smoking, Vape Pen Kits suppliers/factory, wholesale high-quality
products of Modern E-Cigarette R & D and manufacturing, we have the perfect after-sales service and technical support. Look forward to
your cooperation!
maskking high 2.0 vape disposable,maskking high 2.0 electronic cigarette,maskking high 2.0 vape kit,maskking high 2.0 vape starter kit,maskking high 2.0 vape mod kit
Ningbo Autrends International Trade Co.,Ltd. , https://www.supermosvape.com